Math, Science and Pedagogy MCQ Question with Answer | ||||
| Quiz-1 | Quiz-2 | Quiz-3 | Quiz-4 | Quiz-5 |
| Quiz-6 | Quiz-7 | Quiz-8 | Quiz-9 | Quiz-10 |
| Quiz-11 | Quiz-12 | Quiz-13 | Quiz-14 | Quiz-15 |
Q1. The value of 2.48×2.48–1.52×1.520.96 is
(a) 4
(b) 0:96
(c) 16
(d) 15.04
| Answer: (a) 4 Solution:2.48×2.48–1.52×1.520.96 = (2.48+1.52)(2.48−1.52)(2.48−1.52) {Using formula (a2 – b2 = (a+b)(a–b) } = 4 |
Q2. 1f a4=b5=c6 then the value of a+b+cb
(a) 3
(b) 2
(c) 6
(d) 4
| Answer: (a) 3 Solution: a4=b5=c6=k ∴ a = 4k, b=5k, c=6k ∴a+b+cb = 4k+5k+6k5k=15k5k=3 |
Q3. If n is a natural number than √n is
(a) Always a whole number
(b) Always a natural number
(c) Sometimes a natural number and sometimes an irrational number
(d) Always an irrational number
| Answer: (c) sometimes a natural number and sometimes an irrational number Solution: √3, √9=3 Where 9 and 3 are natural number √3is irrational number |
Q4. If √5 =2.24, then the value of 3√52√5−0.48
(a) 1.68
(b) 16.8
(c) 168
(d) 0.168
| Answer: (a) 1.68 Solution: 3√52√5−0.48 = 3×2.242×2.24−0.48=1.68 |
Q5. The greatest fraction out of 25,56,1112and78
(a) 7/8
(b) 5/6
(c) 11/12
(d) 2/5
| Answer: (c) 11/12 Solution: LCM of 8, 6, 12, 5 = 120 Now, 78×120=105; 56×120=100; 1112×120=110; 25×120=48 Clearly greatest fraction is 11/12 |
Q6. The line 3x – 5y = 10 cuts Y-axis at
(a) (0, 2)
(b) (0, 1)
(c) (0. 3)
(d) (0, 4)
| Answer: (a) (0, 2) Solution: at Y-axis x=0, put the value of x in equation 3.0 – 5y = 10 ⇒ 5y = 10 or y = 2 Required point on y-axis (0, 2) |
Q7. If x = 23 x32 x 54 and y = 22 x32 x5 x7, then HCF of x and y is
(a) 180
(b) 360
(c) 540
(d) 35
| Answer: (a) 180 Solution: x = 23 x32 x 54 y = 22 x32 x5 x7 HCF of x and y = Highest common factor of x and y = 22×32×5 = 180 |
Q8. The expression [(√2)√2]√2 gives
(a) a natural number
(b) an integer and not a natural number
(c) a rational number but not an integer
(d) a real number but not a rational number
| Answer: (d) a real number but not a rational number |
Q9. Three numbers are in the ratio 3 : 2 : 5 and the sum of their squares is 1862. What are the three numbers?
(a) 18, 12, 30
(b) 24, 16, 40
(c) 15,10, 25
(d) 21,14, 35
| Answer: (d) 21,14, 35 Solution: Let three numbers are 3k, 2k, and 5k According to question, 9k2 + 4k2 + 25k2 = 1862 ⇒ k2 = 1862/38 = 49 k = 7 Three numbers are 21, 14, and 35 |
Q10. A man sells fans at the same price on one his gain 20% and losses 20% on ‘ the other. His gain or loss is:
(a) 4% loss
(b) 4% gain
(c) Neither gain nor loss
(d) 1% loss
| Answer: (a) 4% loss Solution: In these case always loss = 20×20100=4 |
Q11. A rise of 25% in the price of grapes compels a person to buy 1.5 kg of grapes less for Rs. 240. Then, the original price of grapes per kg is
(a) Rs. 40
(b) Rs. 32
(c) Rs. 30
(d) Rs. 28
| Answer: (b) Rs. 32 Solution: Using tricks, The original price of grapes = 240×25(100+25)×1.5=32 See Trick=> Click Here |
Q12. 7 men and 8 boys can do a piece of work in 2 days. 4 men and 12 boys can do 29/56 of the same work in 1 day. In how many days will 1 man do this work?
(a) 24 days
(b) 25 days
(c) 27 days
(d) 28 days
| Answer: (d) 28 days Solution: Let one man can complete the work in m days and one boys can b days According question, 7m+8b=12 ⇒21m+24b=32 (multiplying 3 both side) —– (i) and 4m+12b=2956 ⇒8m+24b=2928( multiplying 2 both side) —– (ii) we get (i) – (ii) ⇒21m−8m=32−2928 ⇒13m=1328 ⇒ m = 28 |
Q13. A train passes telegraph post in the 40s moving at a rate of 36 km/h. Then, the length of the train is
(a) 400 m
(b) 500 m
(c) 450 m
(d) 395 m
| Answer: (a) 400 m Solution: Speed of train = 36×518=10m/s Length of the train = 40 × 10 = 400 Metre |
Q14. 1n figure determines the value of y.
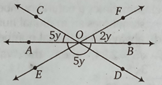
(a) 25°
(b) 35°
(c) 15°
(d) 40°
| Answer: (c) 15° Solution: AOB is straight line, ∠AOC +∠COF +∠FOB = 180 ⇒ ∠AOC +∠COF + ∠EOD = 180 (∠FOB = ∠EOD opposite angle) ⇒ 5y + 5y + 2y = 180 ⇒ y = 15 |
Q15. If the arms of one angle are respectively parallel to the arms of another angle, then the two angles are
(a) Neither equal nor supplementary
(b) Not equal but supplementary
(c) Equal but not supplementary
(d) Either equal or supplementary
| Answer: (b) not equal but supplementary Solution: If the arms of one angle are respectively parallel to the arms of another angle, then the two angles not equal but supplementary
Line l1 || Line l2 then ∠1 ≠ ∠2 but ∠1 + ∠2 = 180° |
Q16.1n the given figure, ABC is a triangle, BC is parallel to AE. If BC = AC, then what is the value of ∠CAE?
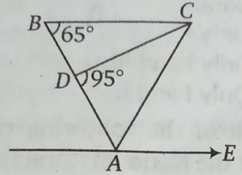
(a) 20°
(b) 30°
(c) 40°
(d) 50°
| Answer: (d) 50° Solution: Given that, BC || AE ∴ ∠CAB + ∠BAE = 180° ∠BAE = 180° – 65° = 115° Again, BC = AC ∴∠CAB = ∠ ABC = 65° ∴∠CAE = 115° – 65° = 50° |
Q17. ABCD is a trapezium in which AB || CD and AB = 2CD. Its diagonals intersect each other at 0, then the ratio of the ‘areas of the Δ A0B and Δ COD is
(a) 1 : 2
(b) 2 : 1
(c) 4 : 1
(d) 1 : 4
| Answer: (c) 4 : 1 Solution:
Let PQ be the perpendicular distance between the two parallel sides AB and CD AB = 2CD, ∴ OP = 2OQ So, AreaofΔAOBAreaofΔCOD = 12×OP×AB12×OQ×CD = 12×2OQ×2CD12×OQ×CD = =41=4:1 |
Q18. In a circle of radius 17 cm, two parallel chords are drawn on the opposite sides of a diameter. The distance between the chords is 23 cm. If the length of one chord is 16 cm, then the length of the other chord is
(a) 34 cm
(b) 15 cm
(c) 23 cm
(d) 30 cm
| Answer: (d) 30 cm Solution:
Here, BE =12AB=162=8cm OB = OD = 17 cm In right angled ΔOEB, OE = √OE2−BE2 = √172−82=15 ∴ OF = EF – OE = 23 – 15 = 8 In right angled ΔOFD, FD = √OD2−OF2 = √172−82=15 ∴ Length of other chord = 2FD = 15×2 = 30 |
Q19. The given graph here shows the production of food grains of a country in different years. Find the percentage 1 increases in production from 1984 to 1985
(a) 15
(b) 30
(c) 50
(d) 100
| Answer: (d) 100 |
Q20. Which one is correct for the following data?
–1, 3, 2, 0, 1 6, 8, 5, 10, 11, 5
(a) Mean = Median = Mode
(b) Mean =Mode
(c) Mode = Median
(d) Mean =5
| Answer: (c) Mode = Median Solution: Given, –1, 3, 2, 0, 1 6, 8, 5, 10, 11, 5 Mean = −1+3+2+0+1+6+8+5+10+11+511=4.54545 Arranging in ascending order, –1, 0, 1, 2, 3, 5, 5, 6, 8, 10, 11 n= 11 Median = n+12 = 11+12 = 6th term Median = 5 Also mode = 5 ∴ Median = mode |
Q21.Which point is not included in the professional skills of a teacher?
(a) Self-knowledge
(b) To experiment well
(c) Dedication
(d) Satisfactory knowledge of the social matter
| Answer: (d) Satisfactory knowledge of the social matter |
Q22. ‘Correlation indicates a joint relationship between two variables.
(a) Ferguson
(b) Lathrop
(c) Skinner
(d) Crowder
| Answer: (b) Lathrop |
Q23. Characteristics of a good textbook of Mathematics should be
(a) there are many solved examples and some questions of exercise
(b) there are sufficient solved illustrations and more questions of exercise
(c) all the exercise questions are solved
(d) All of the above
| Answer: (b) there are sufficient solved illustrations and more questions of exercise |
Q24. ” Mathematics is a way to settle in the mind the habit of reasoning” has been said by
(a) Napoleon
(b) Locke
(c) Socrates
(d) Plato
| Answer: (b) Locke |
Q25. The main function of teaching during the interactive stage is
(a) to realise the size of the class
(b) to diagnose the difficulties
(c) to interact with students
(d) All of the above
| Answer: (d) All of the above |
Q26. Which one of the following is not a characteristic of Mathematics learning?
(a) Change of behaviour
(b) Environmental origin
(c) Goal-direction
(d) All of the above
| Answer: (d) All of the above |
Q27. Which of the following is not an activity-based teaching method?
(a) Demonstration method
(b) Observation method
(c) Heuristic method
(d) Lecture method
| Answer: (d) Lecture method |
Q28. To be good in Mathematics, one needs to
(a) have mastery over calculations
(b) create and formulae problems through abstract thinking and logical reasoning
(c) memorise formulae
(d) remember solutions
| Answer: (b) create and formulae problems through abstract thinking and logical reasoning |
Q29. When introducing mensuration, a teacher writes all the formulae on the board before proceeding further. This reflects that she is following the
(a) deductive approach
(b) experimental approach
(c) practical approach
(d) inductive approach
| Answer: (a) deductive approach |
Q30. A teacher in grade-VI provided each child with a centimetre grid paper and a pair of scissors. She wanted them to explore how two-dimensional shapes can be folded into three-dimensional objects. Which of the following concepts are the students exploring?
(a) Reflection
(b) Nets
(c) Decimals
(d) Rotation
| Answer: (b) Nets |



