Quiz-5: Math and Pedagogy MCQ Questions with Answer

Math and Pedagogy MCQ Question with Answer
Quiz-1	Quiz-2	Quiz-3	Quiz-4	Quiz-5
Quiz-6	Quiz-7	Quiz-8	Quiz-9	Quiz-10
Quiz-11	Quiz-12	Quiz-13	Quiz-14	Quiz-15

Directions (Q. 1–30): Answer the following questions by selecting the most appropriate option.

Q1. Each side of a cube is 3 cm. What will be the area of the cube?

(a) 54 cm³

(b) 36 cm³

(d) 27 cm²

Answer: (c)

Explanation: Volume of cube = s³ = 3³ = 27 cm³

Q2. A book is an example of a

(a) Cube

(b) Cuboids

(d) Square

Answer: (b)

Explanation: As the length, breadth and height are different in a book, it is an example of a cuboid.

Q3. Which of the following points is on the exterior of ∠ ABC?

(a) P

(b) Q

(d) None of these

Answer: (a)

Explanation: Point P is outside of ∠ABC as it lies on the line segment BD.

Q4. ABC is an isosceles triangle with AB = AC. If ∠ A = 40° ∠B =?

(a) 40°

(b) 60°

(d) 50°

Answer: (c)

Explanation: Triangle is an isosceles triangle

Therefore, ∠B = ∠C….. (a)

Sum of the angles of triangle is 180°

∠A + ∠B + ∠C = 180°

40° + ∠B + ∠B = 180° (Given ∠A=40° and using (i) ∠B = ∠C)

2∠B = 180°– 40°

∠B = $\frac{140}{2}=70\degree$

Q5. If Town A has 26547 residents and Town B has 42689 residents, what is the total number of residents in Town A and B together?

(a) 79236

(b) 69236

(d) 68236

Answer: (b)

Explanation: Total number of residents in towns A and B = 26547+ 42689 = 69236

Q6. 5869 – 2864 is equal to

(a) 3005

(b) 2005

(d) 2015

Answer: (a)

Explanation: 5869 – 2864 = 3005

Q7. The difference between n-digit least number and (n–1)-digit greatest number is

(a) 1

(b) 0

(d) – 1

Answer: (a)

Explanation: The difference between n-digit least number and (n–1)-digit greatest number is always 1. Example 1: Four-digit least number = 1000

Three-digit greatest number = 999

Difference = 1000 – 999 = 1

Example 2: Six-digit least number = 100000

Five-digit greatest number = 99999 Difference = 100000 – 99999 = 1

Q8. $3\frac{2}{5}+4\frac{3}{5}+5\frac{1}{5}+3\frac{4}{5}$ is equal to

(a) 15

(b) 17

(d) 20

Answer: (b)

Explanation: $3\frac{2}{5}+4\frac{3}{5}+5\frac{1}{5}+3\frac{4}{5}$

= $3+\frac{2}{5}+4+\frac{3}{5}+5+\frac{1}{5}+3+\frac{4}{5}$

= $3+4+5+3+\frac{2+3+1+4}{5}$

= 15 + 2 = 17

Q9. Which of the following numbers is completely divisible by 11?

(a) 254614

(b) 137016

(d) 620205

Answer: (b)

Explanation: If the difference between the sum of digits at even places and the sum of digits at odd places is either 0 or divisible by 11, the number is divisible by 11.

Sum of digits at odd places in 137016 = 6 + 0 + 3 = 9

Sum of digits at even places in 137016 = 1 + 7 + 1 = 9

Difference = 9 – 9 = 0, which is divisible by 11.

Q10. What is the product of all the factors of 30?

(a) 8100

(b) 81000

(d) 810000

Answer: (d)

Explanation: Factors of 30 are 1, 2, 3, 5, 6, 10, 15 and 30.

∴ 1×2×3×5×6×10×15×30=810000

Q11. 300 cm + 30 m + 3 km = ?

(a) 333 m

(b) 3033 m

(d) 300 m

Answer: (b)

Explanation: 300 cm + 30 m + 3 km = 3 m + 30 m + 3000 m = 3033 m

Q12. Ruhi walks 2 km in 18 minutes. How much time will she take to walk 5 km?

(a) 40 min

(b) 45 min

(d) 55 min

Answer: (b)

Explanation: Time is taken to walk 2 km = 18 min

Time taken to walk 5 km = $\frac{18}{2}\times 5=45$ min

Therefore, to walk 5 km, she will take 45 min

Q13. The weight of a tablet is 3.5 mg. If a company has 17.5 kg of medicine, how many tablets can be made from the medicine?

(a) 5 lac

(b) 50 thousand

(d) 50 lac

Answer: (a)

Explanation: As 1 kg = 100000 mg

∴ 17.5 kg = 1750000 mg

Now, 3.5 mg medicine constitutes 1 tablet

∴ 1 mg medicine constitutes $\frac{1}{3.5}$ tablet

Thus, 1750000 mg medicine $\frac{1}{3.5}\times 1750000=500000$ tablets

Q14. Which of the following statements is correct about measuring balance?

(a) It has three pans of equal weight.

(b) It is used to compare the weight of objects.

(d) It is used to compare the positions of objects.

Answer: (b)

Explanation: A measuring balance is used to compare the weight of objects.

Q15. The next term in the series 1, 1, 2, 6, 15, 31, 56 is

(a) 82

(b) 88

(d) 108

Answer: (c)

Explanation: The difference between the first two numbers is 0 (0²).

The difference between the second two numbers is 1 (1²).

The difference between the third two numbers is 4 (2²).

The difference between the fourth two numbers is 9 (3²).

So, the next number will be 36 more than 56.

Q16. Look at this addition worksheet of a student: What type of error is this?

(a) Incorrect operation

(b) Wrong algorithm

(d) Basic fact error

Answer: (d)

Explanation: It is a basic fact error. The student understands regrouping but commits mistakes in the simple addition of numbers.

Q17. Puzzles like “I have more than 6 tens and less than 5 ones. What number am I?” provide joyful learning in

(a) Counting up to 100

(b) Strengthening the concept of place value

(d) Writing number names

Answer: (b)

Explanation: Such puzzles strengthen the concept of place value in students in an engaging way. They make students think of numbers that satisfy the conditions of the puzzle.

Q18. A teacher of Class II gives the following word problem on ‘subtraction’ to the students to solve: “Vicky had 10 toffees. He gave 5 toffees to his sister. How many toffees are left with Vicky?” Which of the following models/categories does this type of word problem belong to?

(a) Augmentation

(b) Segregation

(d) Aggregation

Answer: (b)

Explanation: This word problem comes under the segregation category because Vicky has given away half of his toffees to his sister, which implies separating from others or from a whole.

Q19. Nishat solved a simple addition sum in the following manner: What type of error is this?

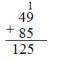
(a) Incorrect operation

(b) Wrong algorithm

(d) Basic fact error

Answer: (b)

Explanation: The wrong algorithm has been applied here. Although Nishat tried well to execute the algorithm, she has not understood the right procedure as yet. The teacher needs to clarify the concept of place value and help her understand, how to do regrouping using appropriate manipulators.

Q20. Ankita writes fifty-six as 506 and twenty-five as 205. What is the learning impediment of Ankita and how can the teacher help her?

(a) Ankita has not understood the concept of place value properly.

(b) Ankita has not yet learnt to count up to 100.

(d) Ankita is a careless girl. She never pays attention to her studies.

Answer: (a)

Explanation: Ankita has not understood the concept of place value properly. The teacher should use a number of manipulators to give her hands-on experiences of learning maths.

Q21. Vishal learns better when taught with the help of beads, marbles, etc. He was taught the concept of division as repeated subtraction using marbles. Vishal

(a) has dyslexia

(b) is a kinaesthetic learner

(d) is a very intelligent child

Answer: (b)

Explanation: Vishal is a kinaesthetic learner. He learns better by touching and doing things using objects. If manipulative like pebbles, beads and blocks are used, he will learn better.

Q22. Mental maths is not

(a) thinking logically

(b) enhancing the understanding level of students

(d) the memorisation of basic mathematical facts, such as knowing the timetables by heart

Answer: (d)

Explanation: Mental maths is not about memorising basic mathematical facts, but provides ways to develop mental computational procedures as the students try to identify the relationship between numbers for faster calculation.

Q23. Different steps of teaching multiplication to Class-III students are given below, but they are not in sequential order.

Select the right order of the steps.

i. Repeated addition using equations (For example, 6 + 6 + 6 = 18)
ii. Multiplication of two single-digit numbers using tables
iii. Multiplication as a short form of repeated addition (For example, 6 + 6 + 6 = 6 × 3 = 18)
iv. Repeated addition through a story

Learning tables

(a) iv, i, v, iii, ii

(b) iv, i, v, iii, ii

(d) iv, ii, iii, i, v

Answer: (b)

Explanation: For effective teaching of multiplication to Class-III students, a teacher should first teach the students about repeated addition through a story, then use equations such as 6 + 6 + 6 = 18. Next, he should teach about how to work with tables, and later about multiplication as a short form of repeated addition such as 6 + 6 + 6 = 6 × 3 = 18 and lastly about the multiplication of two single-digit numbers using tables.

Q24. Building a mathematical wall in the classroom helps students

(a) understand difficult concepts in mathematics

(b) know their position in the class

(d) share their views and problems with others and show their creative ability

Answer: (d)

Explanation: Mathematical walls are an important part of the classroom. Students take ownership and share their ideas. It is more than just words, and students improve their maths vocabulary.

Q25. A teacher is observing students working in groups. Her observation is focused on collaboration and cooperation in the group, the concentration and interest of each student and the participation of the individual students in the activity. Through this activity, she is trying to

(a) do a summative assessment of the students

(b) do formative assessment of the students

(d) give training to the students in life skills

Answer: (b)

Explanation: The teacher is doing a formative assessment. Options (c) and (d) are part of the formative assessment itself. So, option (b) is the most appropriate answer.

Q26. Which one of the following is not a problem-solving strategy in mathematics?

(a) Rote learning

(b) Trial and error

(d) Solving backwards

Answer: (a)

Explanation: Rote learning is not a problem solving strategy in mathematics. It is because mathematics is a concept-based subject that makes use of numbers, calculations and real-life situations.

Q27. Read the following problem given in a textbook for Class V: A map is given with a scale of 2 cm = 1000 km. What is the actual distance between two places, in km, if the distance on the map is 2.5 cm? The problem is

(a) to enhance problem-solving skills

(b) interdisciplinary in nature

(d) based on higher-order thinking skills

Answer: (b)

Explanation: In mathematics teaching, when a problem involves two or more aspects within a calculation or study, it is interdisciplinary in nature. The given question also has two aspects, i.e., ‘scale value’ and ‘calculate actual value’. Therefore, it is interdisciplinary.

Q28. The term ‘mathematical‘ refers to

(a) calculators, rulers, tape measures, protractors, compass, etc.

(b) all types of materials including language, written symbols, meaningful instructions to establish their purpose

(d) charts based on formulae and concepts, graph papers, dotted sheets, etc.

Answer: (b)

Explanation: The term ‘mathematical’ includes all types of materials including language, written symbols and meaningful instructions to establish their purpose. Mathematics is a conceptual subject that is based on data, facts, reasoning and logical thinking, etc.

Q29. “It is more useful to know how to mathematize than to know a lot of Mathematics.” This statement is given by

(a) David Wheeler

(b) George Pólya

(d) Vygotsky

Answer: (a)

Explanation: David Wheeler gave this statement to propose that mathematics is a subject that does not only promote thinking but also the ability to handle abstractions. Its teaching should be such that students learn to solve mathematical or calculative problems with the right attitude as and when required.

Q30. A teacher asked the students to collect leaves and to identify symmetry patterns. This task reflects the teacher’s efforts to

(a) relate real-life experiences with mathematical concepts

(b) introduce an interdisciplinary approach

(d) improve mathematical communication

Answer: (a)

Explanation: Such a type of task reflects the teacher’s efforts to make the students able to connect the learning experiences gained in the class to the real world. In this way, they will develop math skills for use in real situations and may even get more enthusiastic about the subject. In Bloom’s Taxonomy, such kinds of activities have been referred to as the ‘analysis level’.

For Latest Post Join our Telegram Channel

Quiz-5: Math and Pedagogy MCQ Questions with Answer

Math and Pedagogy MCQ Question with Answer

Read Important Article

Leave a Comment Cancel reply

© Copyright 2020: Exam Portal