CTET January 2012 Question Paper-1 with Answer | ||||
| Child Development | Mathematics | EVS | Language-I (Eng) | Language-II (Hindi) |
Mathematics
Direction: Answer the following questions by selecting the most appropriate option.
- A Pencil costs two and a half rupees. Amits buys one and one half dozen pencils and gives a 100 rupee note to the shopkeeper. The money he will get back is:
(a) Rs. 30
(b)Rs. 55
(c) Rs. 45
(d) Rs. 65
| Answer: (b) Rs. 55 Sol: Cost of pencil = 2.50 Amit bought one and one half dozen pencils = 12+6= 18 Total Cost of pencils = 18 *2.50 = Rs. 45 The money he will get back is = 100-45 = Rs. 55 |
- Which of the following is correct?
(a) The successor of a predecessor of 1000 is 1001
(b) The successor of a predecessor of 1000 is 1002
(c) A predecessor of the successor of 1000 is 1000
(d) A predecessor of the successor of 1000 is 999
| Answer: (c) Predecessor of the successor of 1000 is 1000 Sol: successor of 1000 = 1000+1=1001 then predecessor of 1001 = 1000-1 = 1000 |
- A shop has 239 toys. Seventy more toys are bought in then 152 of them were sold. The number of toys left was
(a) 239 +70 – 152
(b) 239 – 70 – 152
(c) 239 + 70 + 152
(d) 239 – 70 + 152
| Answer: (a) 239 +70 – 152 Sol: Total toys: 239 bought 70 toys then total = 239 + 70 after that sold 152 The number of toys left was = (a) 239 +70 – 152 |
- In the product 3759 × 9573, the sum of tens digit and the unit digit is
(a) 16
(b) 7
(c) 0
(d) 9
| Answer: (b) 7 Sol: product 3759 × 9573 = 35984907; ten’s digit 0 and unit’s digit 7 sum of them = 0+ 7 = 7 |
- While planning a lesson on the concept of fraction addition, a teacher is using the activity of strip folding:
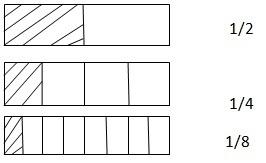
The above activity is a
(a) wastage of time
(b) pre-content activity
(c) content activity
(d) post-content activity
| Answer: (b) Before teaching a lesson, pre-content activity helps students to understand and learn easily. |
- A suitable approach for explaining that a remainder is always less than the divisor to class IV students can be
(a) represent division sums as mixed fractions and explain that the numerator of the fraction part is the remainder
(b) grouping of objects in multiples of the divisor and showing that number the number of object s, not in the group is less than the divisor
(c) perform lots of division sums on the blackboard and show that every time the remainder is less than the divisor
(d) explain verbally to the students, several times
| Answer: (b) grouping of objects in multiples of the divisor and showing that number the number of object s, not in the group are less than the divisor |
- In which of following divisions, will the remainder be more than the remainder you get when you divide 176 by 3?
(a) 176 ÷ 2
(b) 173 ÷ 5
(c) 174 ÷ 4
(d) 175 ÷ 3
| Answer: (b) 173 ÷ 5 Sol: Remainder when divide 176 by 3 = 2 And from option when we divide 176 ÷ 2, remainder = 0 173 ÷ 5, remainder = 1 174 ÷ 4, remainder = 2 175 ÷ 3, remainder = 3 |
- Rizul is a kinesthetic learner. His teacher Ms. Neha understood his style of learning. Which of the following strategies should she choose to clear his concept of multiplication?
(a) Use strings and beads of two different colors to get the multiples of 2,3 etc.
(b) Skip counting
(c) Counting the points of intersection on criss-cross lines
(d) Forcing him to memorize all tables
| Answer: (c) Counting the points of intersection on criss-cross lines |
- Use of Abacus in Class-II does not help the students to
(a) Write the numeral equivalent of numbers given in words
(b) attain perfection in counting
(c) understand the significance of place value
(d) read the numbers without error
| Answer: (a) Write the numeral equivalent of numbers given in words |
- 500 cm + 50 m + 5 km =
(a) 5055 m
(b) 55 m
(c) 500m
(d) 555m
| Answer: (a) 5055 m Sol: 500 cm = 5 m, 5 km = 5000 m, 5 m + 50 m + 5000 m = 5055 m |
- ‘ Recognition of patterns and their completion’ is an essential part of the Mathematics curriculum at the primary stage as it
(a) helps the students in solving ‘ Sudoku’ puzzles
(b) promotes creativity among the students and helps them to understand the properties of numbers and operations
(c) develops creativity and artistic attributes is students
(d) prepares students to take up competitive examinations
| Answer: (b) promotes creativity among the students and helps them to understand the properties of numbers and operations |
- Sum of place values of 6 in 63606 is
(a) 6066
(b) 18
(c) 60606
(d) 6606
| Answer: (c) 60606 Sol: Place values of all 6 in
|
- The difference of 5671 and the number obtained on reversing its digit is
(a) 7436
(b) 3906
(c) 4906
(d) 3916
| Answer: (b) 3906 Sol: Given number = 5671 and the number obtained on reversing its digit is 1765 Difference 5671 – 1765 = 3906 |
[ Read Also: 2011 June CTET Paper-II Questions with Answer ]
- Study the following pattern :
1 × 1 = 1
11 × 11 = 121
111 × 111 = 12321
…………………
…………………
What is 11111 × 11111 =?
(a) 123454321
(b) 1234321
(c) 123453421
(d) 12345421
| Answer: (a) 123454321 |
- How many 1/8 are in ½?
(a) 2
(b) 16
(c) 8
(d) 4
| Answer: (d) 4 Sol: let x times 1/8 are in ½ X*1/8 = ½ ⇒ x = 8*1/2 = 4 |
- 19 thousand + 19 hundred + 19 ones are equal to
(a) 19919
(b) 191919
(c) 21090
(d) 20919
| Answer: (d) 20919 Sol: 19000 + 1900 + 19 = 20919 |
- What time is 4 hours 59 minutes before 2 : 58 P.M?
(a) 9 : 59 P.M
(b) 9 : 57 P.M
(c) 9 : 59 A.M
(d) 9 : 57 A.M
| Answer: (c) 9 : 59 A.M Sol : 2 : 58 P.M – 4 hours 59 minutes = 14 hr 58 min – 4 hr 59 min = 9 hr 58 min = 9 : 58 A.M |
- If the 567567567 is divided by 567, the quotient is
(a) 1001001
(b) 3
(c) 111
(d) 10101
| Answer: (a) 1001001 |
- Ms. Reena uses a grid activity to teach the concept of multiplication of decimals. A sample illustrated below :
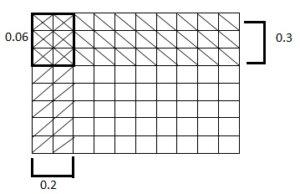
Through this method, Ms. Reena is
(a) focusing on developing problem-solving skill
(b) focusing more on procedural knowledge and less on conceptual knowledge
(c) focusing more on conceptual knowledge and problem solving and less on procedural knowledge
(d) using traditional approach learning
| Answer: (c) focusing more on conceptual knowledge and problem solving and less on procedural knowledge |
- To assess the students’ competency on solving of word problems based on addition and subtraction, rubrics of assessment are
(a) identification of the problem, performing the correct operation
(b) incorrect, partially correct, completely correct
(c) comprehension of the problem, identification of operation to be performed representation of problem mathematically, solution of problem and presentation of the problem
(d) understanding of problem and writing of correct solution
| Answer: (c) comprehension of the problem, identification of operation to be performed representation of problem mathematically, solution of problem and presentation of the problem |
- The solid as shown in the figure is made up of cubical blocks each of side 1 cm. The number of the block is

(a) 72
(b) 48
(c) 52
(d) 60
| Answer: (d) 60 Solution: Numbers of block = 3 × 4 × 5 = 60 |
- The NCF (2005) considers that Mathematics involves ‘a certain way of thinking and reasoning’. The vision can be realized by
(a) rewriting all text-books of Mathematics
(b) giving lots of problem worksheets to students
(c) giving special coaching to students
(d) adopting the exploratory approach, use of manipulative, connecting concepts to real life, involving students in discussions
| Answer: (d) adopting the exploratory approach, use of manipulative, connecting concepts to real life, involving students in discussions |
- The figure consists of five squares of the same size. The area of the figure is 180 square centimeters. The perimeter (in cm) of the figure will be
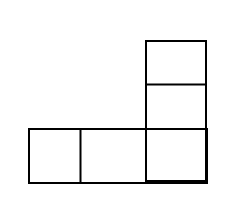
(a) 72
(b) 36
(C) 45
(d) 48
| Answer: (a) 72 Solution: Area of given figure = 180 cm2 , the figure consist 5 square So, area of one square = 180/5 = 36 cm2 , side of square = cm perimeter (in cm) of the figure = 2 × 3 × 6 + 2 × 2 × 6 + 2 × 6 = 72 cm |
- While teaching the addition of fractions, it was observed by Mr. Singh that the following type of error is very common: 23+25=410
Mr. Singh should take the following remedial action:
(a) Advise the students to work hard and practice the problems of fraction addition
(b) Explain the concept of LCM of the denominator
(c) Give more practice of the same type of problems
(d) Give pictorial representation to clear the concept of addition of unlike fractions, followed by a drill of the same type of problems
| Answer: (d) Give pictorial representation to clear the concept of addition of unlike fractions, followed by the drill of the same type of problems |
- The most appropriate strategy that can be used to internalize the skill of addition of money is
(a) Solving lots of problems
(b) Use of ICT
(c) Use of models
(d) Roleplay
| Answer: (d) Roleplay |
- A teacher uses the following riddle in a class while developing the concept of base 10 and place value I am less than 8 tens and 4 ones. The objective of this activity is
(a) to do a summative assessment
(b) to introduce the concept of tens and ones to the students
(to have some fun in the class and to break the monotony
(d) to reinforce the concept of base 10 and place value
| Answer: (d) to reinforce the concept of base 10 and place value |
- The objective of the teaching number system to Class III students is to enable the students
(a) to master the skill of reading large numbers
(b) to count up to 6 digits
(c) to see the numbers as groups of hundreds, tens and ones and to understand the significance of place value
(d) to master the skill of addition and subtraction of four-digit numbers
| Answer: (c) to see the numbers as groups of hundreds, tens and ones and to understand the significance of place value |
- The concept of areas of plane figures can be introduced to the students of Class V by
(a) stating the formula for the area of rectangle and square
(b) calculating the area of figures with the help of counting unit squares
(c) measuring the area of any figure with the help of different objects like palm, leaf, pencil, etc.
(d) calculating the area of a rectangle by finding the length and breadth of a rectangle and using the formula for the area of a rectangle
| Answer: (c) measuring the area of any figure with the help of different objects like palm, leaf, pencil, etc. |
- Computational skills in Mathematics can be enhanced by
(a) clarifying concepts and procedures followed by lots of practice
(b) giving conceptual knowledge alone
(c) describing algorithm only
(d) conducting hands-on activities in class
| Answer: (d) conducting hands-on activities in class |
- To teach various units of length to the students of Class III a teacher shall take the following materials to the class
(a) Measuring tape with centimetre on one side and meter on the other side
(b) Relation chart of various units
(c) Centimetre ruler and measuring tape
(d) Rulers of different lengths and different units measuring rod, measuring strip used by architects
| Answer: (d) Rulers of different lengths and different units measuring rod, measuring strip used by architects |

