2011 CTET Paper-1 Question with Answer
| Child Development | Mathematics | Environment studies | Language-I(Eng) | Language-II(Hindi) |
MATHEMATICS
Directions: Answer the following questions by selecting the most appropriate option.
31. While teaching a comparison of fractions which the numerators are the same e.g. 3/5 and 3/7 Rohit’s response was “since the numerators are same and since 7 is larger than 5, therefore 3/7 is bigger than 3/5 .” This suggests that
(a) Rohit does not know the concept of equivalent fractions
(b) Rohit has not practiced well
(c) Rohit does not understand the magnitude of fractions
(d) Rohit does not know the concept of numerator and denominator
32. Examine the following matchstick patterns:

If the pattern continues, how many matchsticks are needed in the 15th stage?
(a) 61
(b) 62
(c) 105
(d) 65
33. To introduce the concept of fractions, a teacher can begin with
(a) writing fractions in the form of a/b where b ≠ 0
(b) identifying fractional parts of things around them
(c) identifying numerators and denominators of different fractions
(d) finding fractions on a number line
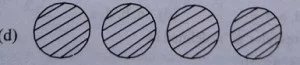
34. ![]() is 3/4 of a ‘unit’. What will be 112?
is 3/4 of a ‘unit’. What will be 112?

35. The number 49532 rounded off to the nearest thousand is
(a) 41000
(b) 50000
(c) 49000
(d) 49500
36. In the following, which is the greatest number?
(a) [(2+2)2]2
(b) (2+2+2)2
(c) (d)2
(d) (2×2×2)2
37. “Start a discussion in the class on things in the child’s environment which roll and slide. Help children to look at their shapes and see how some things roll and others slide.”Suggestions like this have been given in the NCERT textbook of Class-II to help a teacher understand that
(a) discussions supplemented with demonstration help students to understand concepts better
(b) discussions bring multiple perspectives into the classroom
(c) discussion is the best strategy for the mathematics classroom
(d) it is imperative for the teachers to draw the children’s attention to the things around them
38. The chapters in the NCERT textbook of mathematics of Class-IV have titles like 38. “The Junk Seller”, “Trip to Bhopal”, “The Way the World Looks”. This shift has been done to
(a) make it interesting by relating it to everyday life
(b) know about selling junk and traveling
(c) challenge the students to guess the mathematical content in the chapters
(d) make them understand differently
39. The weight of some mangoes is 2 kg 600 g and that of some apples is 1 kg 450 g. The weight of the mangoes is greater than that of the apples by
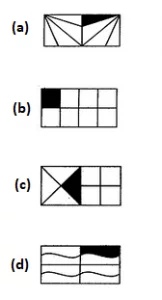
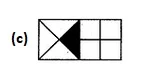
(a) 1 kg 200 g
(b) 150 g
(c) 4 kg 50 g
(d) 1 kg 150 g
40.”Problem solving” as a strategy of doing mathematics involves
(a) activity based approach
(b) estimation
(c) extensive practice
(d) using clues to arrive at a solution
41. Sequence the following tasks as they would be taken up while. developing the understanding of shapes and space across primary classes :
a. Matches the properties of 2-D shapes by observing their sides and corners
b. Describes intuitively the properties of 2-D shapes
c. Sorts 2-D shapes
d. Describes the various 2-D shapes by counting their sides, corners, and diagonals
(a) a, d, b, c
(b) c, a, d, b
(c) d, b, a, c
(d) c, b, d, a
42. If an operator ⬲ is defined as
4 ⬲ 3 = ᷿4 + 5 + 6
5 ⬲ 4 = 5 + 6 + 7 + 8
6 ⬲ 4 = 6 + 7 + 8 + 9
what will n ⬲ 8 be equal to ?
(a) 8n + 36
(b) n + 36
(c) n + 28
(d) 8n + 28
44. The purpose of a diagnostic test in mathematics is
(a) to fill the progress report
(b) to plan the question paper for the end-term examination
(c) to know the gaps in children’s understanding
(d) to give feedback to the parents
45. 407928 is read as
(a) Forty thousand nine hundred twenty-eight
(b) Four lakh seven thousand nine hundred twenty-eight
(c) Four lakh seventy-nine thousand twenty-eight
(d) Forty-seven thousand nine hundred twenty-eight
46. The length of a rectangle is ‘l’ and its width is half of its length. What will be the perimeter of the rectangle if the length is doubled keeping the width the same?
(a) 6l
(b) 3l
(c) 4l
(d) 5l
47. Which is true for a hexagonal pyramid?
(a) It has two hexagonal faces and six rectangular faces
(b) It has six hexagonal faces joined by six rectangular faces
(c) It has six faces and each face is a hexagon
(d) It has a hexagonal base with six triangular faces meeting at a point
48. How many 4-digit numbers are there in the Hindu-Arabic Numeration System?
(a) 9999
(b) 9000
(c) 99
(d) 8999
49. Vikas teaches mathematics to a class of 56 students. He believes that conducting a test is effective if the feedback is given immediately. He conducted a short class test of 10 marks. What is the best possible way of giving feedback effectively?
(a) He can have a whole class discussion on ways in which they have got their solutions and which is the effective strategy to arrive at the correct answer
(b) Pick out any copy at random and discuss the method followed in the copy on the board
(c) He can let the students check each other’s answer
(d) He can explain the solution of each problem on the board and ask the students to check their answer on their own
50. When teaching ‘shapes’, a teacher can plan a trip of historical places as
(a) field trips have been recommended by CBSE, so they are a must
(b) shapes are an integral part of any architecture and such trips encourage connections across disciplines
(c) she has completed most of the syllabus well in time and needs to provide leisure
(d) it would be a good break from the routine mathematics class and an opportunity to improve communicative skills
51. In a dice, the numbers on the opposite faces add up to 7. Which among the following will fold into a dice?
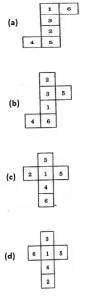



60. To be a “good” mathematician one must be able to
(a) understand, apply and make connections across the concepts
(b) master the techniques of answering questions
(c) memories most of the formulae
(d) solve the problem in no time
