CTET 2011 Question Paper-2 with Answer | ||||
| Child Development | Mathematics and Science | Social studies | Language-I(Eng) | Language-II(Hindi) |
Q51. The above represents the work of a student. If this error pattern continues, the students answer to 5/11 – 2/7 will be
(a) 7/18
(b) 3/4
(c) 3/7
(d) 2/18
| Answer: (b) 3/4 Solution: After subtraction, the student writes the difference of numerator in the numerator of the resultant fraction denominator in the denominator of the resultant fraction. The difference between 5 and 2 =3 The difference between 11 and 7 = 4 Resultant fraction found by the student = 3/4 |
Q52. A teacher in Grade VI provided each child with a centimeter grid paper and a pair of scissors. She wanted them to explore how two-dimensional shapes can be folded into three-dimensional objects. Which of the following concepts are the students exploring?
(a) Rotation
(b) Reflection
(c) Nets
(d) Decimals
| Answer: (c) Nets Explain: The students are exploring the concept of nets. Each will draw a pattern on the centimeter grid paper. They may use a pair of scissors to cut out the shape. After drawing the pattern, they will fold the paper to get a 3-D object. |
Q53. When doing exponents, the work observed in a learner’s notebook was as follows:
43 x 42 = 45
64 x 64 = 68
73 x 37 = 2110
The learner has not understood how to
(a) add exponents
(b) add exponents and multiply
(c) multiply numbers with the same base
(d) multiply numbers with different bases
| Answer: (d) multiply numbers with different bases Explain: The learner Correctly multiplied numbers with the same have a base, but he/she is not able to multiply numbers With different bases. |
Q54. Teachers, while discussing problem-solving as an approach to the teaching of mathematics, articulated four views. Which of the following views does not justify the real meaning of this approach?
(a) I think questions on problem-solving should be made from situations based on real-life.’
(b) I think many questions found in the mathematics textbook can be used for problem-solving
(c) I think it is better to connect problem-solving with general mathematics class.
(d) I think there is no correlation between problem- solving and mathematical reasoning’
| Answer: (d) I think there is no correlation between problem- solving and mathematical reasoning’ Explain: Problem-solving depends mainly on mathematical reasoning They are highly correlated. So, the statement that there is no correlation between problem- solving and mathematical reasoning does not Justify the real meaning of the problem-solving approach. |
Q55. Given linear equations, I, II and III, a learner is not able to solve II algebraically. The most likely area of difficulty Is that the learner has not understood

(a) that two equations can be added or subtracted to Solve them
(b) that two equations can be solved by the method of substitution
(c) the method of solving equations using graphs
(d) that both the equations can be altered by multiplying with suitable numbers
| Answer: (a) that two equations can be added or subtracted to Solve them Explain: Equations given in I and II have the same number of y’s, so they can be subtracted to find x and then y. This is not very apparent in the case where the numbers of x’s and y’s are different. The student does not know how to make them equal. |
Q56. When introducing menstruation, a teacher writes all the formulae on the board before proceeding further. This reflects that she is following the
(a) inductive approach
(b) deductive approach
(c) experimental approach
(d) practical approach
| Answer: (b) deductive approach Explain: Give the formula first, and the students are expected to solve the problems by applying the formula. For better understanding and logical way of solving mensuration problems, the teacher may help the students to justify (prove) the formulae with the help of examples. |
Q57. Ameena is playing with matchsticks and adds one
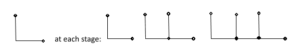
Appu, on the other hand, makes a table:
| Number of L’s | 1 | 2 | 3 | ……. |
| Number of matchsticks | 2 | 4 | 6 | ……. |
What is your observation of two children in this situation?
(a) Ameena is only playing and Appu is doing mathematics.
(b) Ameena will need lots of matchsticks to come to a generalization. However, Appu would be a taster.
(c) Both Ameena and Appu are trying to make generalizations.
(d) Ameena would be learning by doing and Appu may not be able to see the pattern at al
| Answer: (a) Ameena is only playing and Appu is doing mathematics. Explain: Ameena is only playing. She is simply adding 2 matchsticks to the L shape at a time, but Appu is doing mathematics. He is recording the number of matchsticks used by Ameena at every step. |
Q58. To be good in mathematics, one needs to
(a) remember solutions
(b) have mastery over calculations
(c) create and formulate problems through abstract thinking and logical reasoning
(d) memorize formulae
| Answer: (c) create and formulate problems through abstract thinking and logical reasoning Explain: To be good in mathematics, one needs to create and formulate problems through abstract thinking and logical reasoning remembering solutions, memorizing formulae by rote and having mastery over calculations all lack logical thinking and proper understanding. So, they do not make a person proficient in mathematics. |
Q59. Students make errors while solving mathematical problems because
(a) they do not practice enough
(b) they do not refer to multiple textbooks
(c) their Socio-economic status affects their performance
(d) they make alternative interpretations of concepts in their attempt to device meaning
| Answer: (d) they make alternative interpretations of concepts in their attempt to device meaning Explain: When students find difficulty in solving a mathematical problem, they make alternative interpretations of concepts in their attempt to derive meaning This often results in errors. |
Q60. With activity on paper folding, a teacher was trying to depict the relationship of the areas of a parallelogram and a triangle. Which of the following best depicts the transformation of stages?
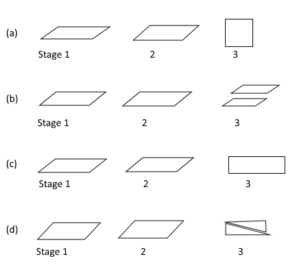
| Answer: (d) The transformation shown in (d) is the best as it is simple, easy to demonstrate just one step) and clearly shows two triangles. |
